In search of the shape of the Universe - the Poincaré Conjecture

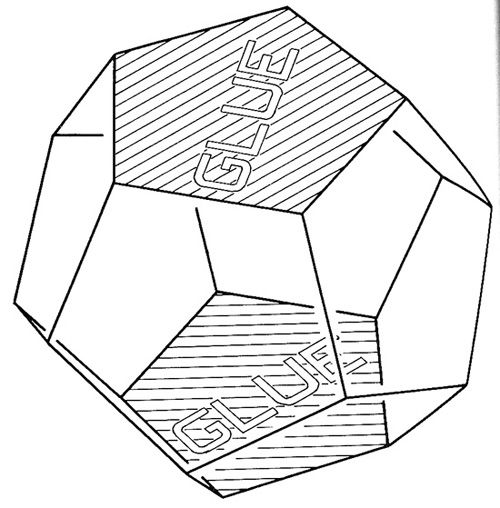
The Poincaré dodecahedral space (above) and is described as the three-manifold we get by gluing together opposite faces of a regular dodecahedron after a one tenth counter-clockwise turn, this makes a closed surface bounding a solid. This is the fifth and last Platonic solid. Pythagoreans would be delighted.

Posed in 1904 by Henri Poincaré, the leading mathematician of his era and the most gifted of all time, the Poincaré conjecture is a possible description of the potential shape of our own Universe. The challenge of proving it has absorbed all of mathematics.

Grigory Perelman, Russian mathematician, gave his revelatory lecture in Cambridge Massachusetts, April 7, 2003, where he invited attendees to imagine our Universe as an element in the gigantic abstract mathematical set of all possible universes.

Trying to pair up different regions in the sky with one another leads to a gigantic statistical problem called cosmic crystallography. Closed loops that cannot be shrunk to a point would show up as spikes in the pair separation histogram, a mathematical loop used to search for periodicity in data. Should the universe be small enough, the absence of such spikes would suggest our universe is simply connected. The first years of the 21st century witnessed a search for the circles that would indicate the universe has the topology of Poincaré's dodecahedral space. The circles were not present. Either the universe is too big, or its fundamental group is different altogether, or, noise may obscure the circles.

The mathematics to determine Poincaré's conjecture included Betti numbers; geometrization conjecture; homeomorphism; Ricci flow; Riemann curvature tensor and topological equivalent. These facets are too advanced for this overview.

We do not know the size or shape of our expanding universe, because we cannot observe it. The night sky with distant stars, galaxies and clusters of galaxies, must contain other intelligent life, some much different from us. When we meet and communicate with we will discover what they know, or, want to know, that the only compact three-dimensional manifold in which every loop can be shrunk to a point is a three-sphere. Be certain this will happen.

Source: The Poincaré Conjecture, In search of the Shape of the Universe, Donal O'Shea, Penguin, 2007